
As a final example in this introduction, here is how Geometer might be used to help solve a real problem. (The problem here would not be considered "research" by a professional mathematician, but if you don't know how to solve it, it's research for you.)
Here's the problem: Given the lengths of the three medians of a triangle, find (in other words, construct) the triangle itself. Let's see how Geometer might help. Recall that a median of a triangle is the line segment connecting a vertex with the midpoint of the opposite side.

First, look at the figure above. We are given the three lengths AA', BB', and CC' and we wish to find the triangle. We know that the point M where the three medians meet is 2/3 of the way from each vertex to the opposite side---in other words, AM = 2A'M, BM = 2B'M, and CM = 2C'M. So if we start with, say, AA', we can find a point M on it that is 1/3 of the way between A' and A (this is a standard construction).
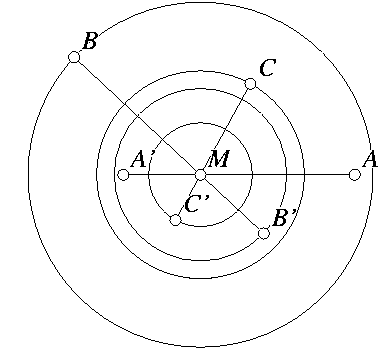
Now look at the figure above. If you know the location of segment AA', you can find where M is, and since you know that all the medians meet at M, and that they are all 2/3 of the distance between the vertex of the triangle and the opposite edge, the points B, B', C, and C' must all lie somewhere on the circles shown in the figure.
So if we leave AA' fixed and move B around its circle to all of its possible positions, we know that the median property will be satisfied if the midpoint between B and A is at C'. (If this isn't clear, look back at the first medians figure and read it again.)
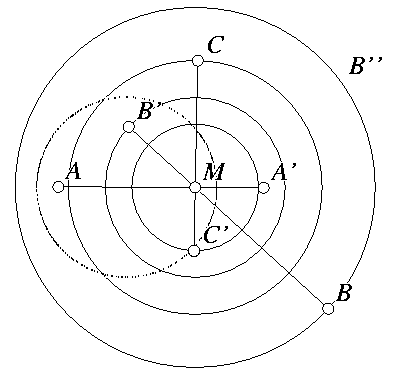
So as B moves around its circle to all of its possible positions, what path does the midpoint between B and A follow? If you're not sure, the best bet is to make that midpoint, draw it in a smearing color, and then move B'' once around its circle (leave B fixed so the circle size doesn't change, and then move B'' around to try all the positions on that circle) to see what you get. What you get is the figure above.
The path of the midpoint of AB appeared to be a circle! Suspecting that this path is, in fact, a circle (and knowing some geometry), it is easy to show that the path of the midpoints is a circle, and to work out the center and radius of that circle. If you construct that circle and the circle of possible locations for C', the point C' has to be at the intersection of those two circles. Once you know where C' goes, it's easy to find C (opposite M from C', and twice as far away), and then B will be on the line CA', and as far from A' as C is.
You could probably have figured this out without Geometer, but it is certainly a lot easier to play with the drawing for a few seconds and notice the critical property for the construction.